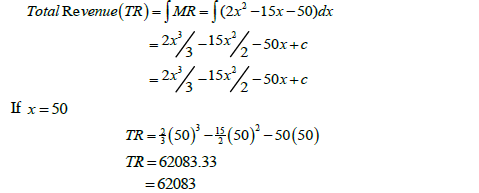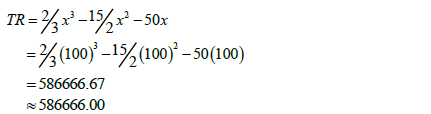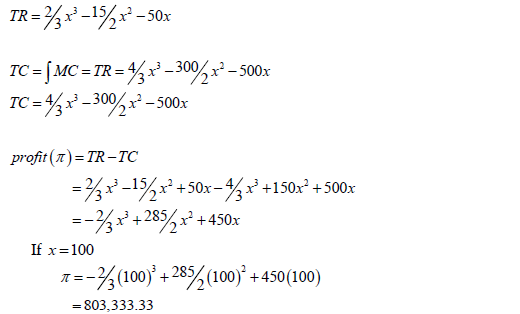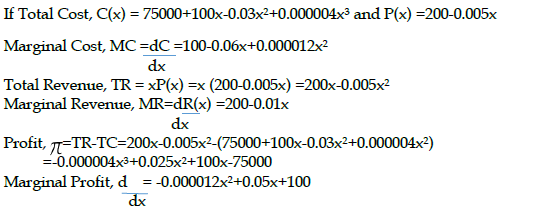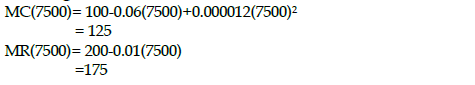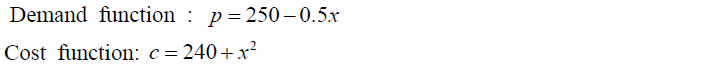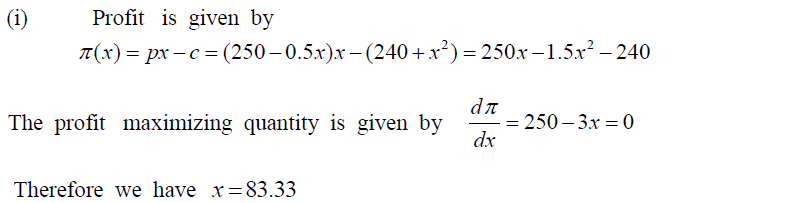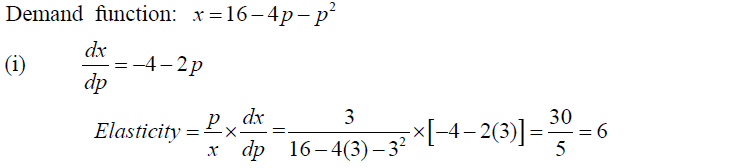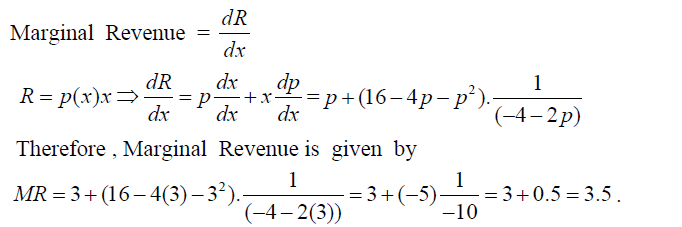a) What is a Stationary Point of a differentiable function of one variable?                                                                        (2 marks)

b) Renes Trading Company sells q kente strips per month at p Ghana Cedis per Kente strip. The demand function for kente strips is given by p=300−0.02q. The kente strips cost GH¢30 per strip to manufacture. There are fixed costs of GH¢9,000 per month.
Required:
i) Determine the price per kente strip that will maximize revenue.                                                                                    (4 marks)

ii) Determine the quantity where profit is maximized.                                                                                                       (4 marks)

iii) Calculate the maximum profit.                                                                                                                                      (2 marks)

c) Due to changes in market conditions, the company finds the demand q, in thousands, for their kente strips to be at a price of GH¢p (p Ghana Cedis).
Required:
i) Determine the elasticity of demand when the price is GH¢5 and when the price is GH¢15 per kente strip.                  (6 marks)

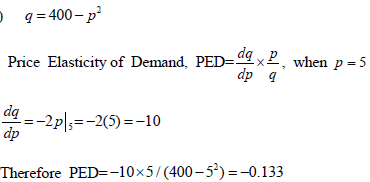
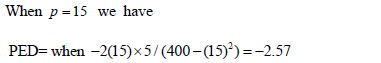
ii) Comment on your results in (i).                                                                                                                                      (2 marks)

The marginal revenue function of a manufacturing company is given by: The marginal cost function is given by:  Let x be the number of items either produced or sold.
Required:
i) Calculate the revenue generated when 50 items are sold.                                                                                              (2 marks)

ii) Calculate the number of items that will yield maximum revenue.                                                                                (4 marks)

iii) Calculate the total revenue if 100 items are produced.                                                                                                (4 marks)

iv) Calculate the total profit for the 100 items.                                                                                                                  (4 marks)

v) If the tax of 20% is imposed on each item produced, find the cost of 100 items.                                                         (6 marks)

a) Distinguish between marginal cost and average cost in production.                                                                               (2 marks)

The company has set production limit to 10,000 Cans and it sells all the Cans that are produced.
Required:
i) Derive an expression for marginal cost, marginal revenue and marginal profit.                                                             (4 marks)

ii) Determine the cost, revenue and profit when the 2501st Can is produced and sold.                                                     (3 marks)

iii) Determine the cost, revenue and profit when the 7501st Can is produced and sold.                                                    (3 marks)

iv) Advise the company whether to produce the 2501st Can or the 7501st Can.                                                                (2 marks)

c) If, after expanding its facilities, the company is capable of producing 60,000 Cans in a day and the total daily cost is given by

Required:
How many Cans per day should they produce in order to minimize production costs?                                                     (6 marks)

a) The AXM manufacturing company has determined that the cost function for producing a particular type of pavement block is given by:

where x is measured in number of units and C(x) in GH¢.

Required:
i) Calculate the derivative of C(x) with respect to x .                                                                                                          (5 marks)

ii) Determine how quickly the cost is changing at x =1000.                                                                                               (4 marks)

b) At the Zee manufacturing company, the marginal cost for producing x gears, measured in hundreds is

MC =10+0.1x .
If the fixed cost (the cost of producing zero items) is GH¢3000.
Required:
Determine the cost for manufacturing 5000 gears.                                                                                                            (11 marks)

a) Sketch Graph(s) to show the following critical points on a cost or revenue function:
i) Local Maximum Point                                                                    (1 mark)
ii) Absolute Maximum Point                                                             (1 mark)
iii) Local Minimum Point                                                                  (1 mark)
iv) Absolute Minimum Point                                                             (1 mark)
v) Point of inflexion                                                                           (2 marks)

b)

If the fixed market price of low grade steel is GH¢ 6.90 and the fixed market price of high grade steel is GH¢ 13.80.
Required:
i) Determine the number tons of low grade steel and high grade steel to be produced to maximize total revenue.            (10 marks)

ii) Determine the maximum total revenue.                                                                                                                         (4 marks)

Required:
i) Calculate, the total cost, average cost, and marginal cost of producing 5000 items.                                                       (6 marks)

ii) Determine the production level at which the average cost will be the lowest.                                                               (4 marks)

Required:
(i) Determine, how many sets per week should he produce to maximize his profit.                                                         ( 5 Marks)

(ii) Determine, the maximize profit.                                                                                                                                   (2 Marks)

(c) The price p and quantity x of a product, Kaako are related by the relation:

Required:
(i) Determine the elasticity of demand at p= 3 .                                                                                                                 (4 Marks) 

(ii) Determine, marginal revenue at p= 3 .                                                                                                                          (4 Marks)